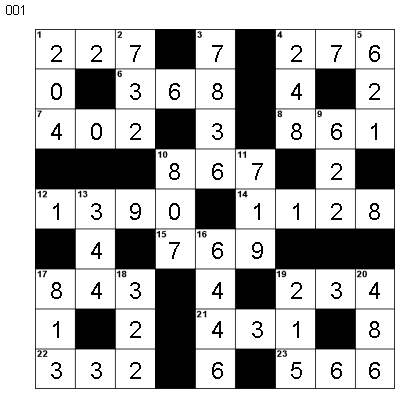
1) Numbers 04, 09, and 16 go in the corners, and the sum of elements in the first column is less than 15. Thus, 16 can't go in A4. If 09 went in A4, then because A2 + A3 is at least 5, the sum of numbers in column 1 would have to be 15 or more. Thus, A4 = 04.
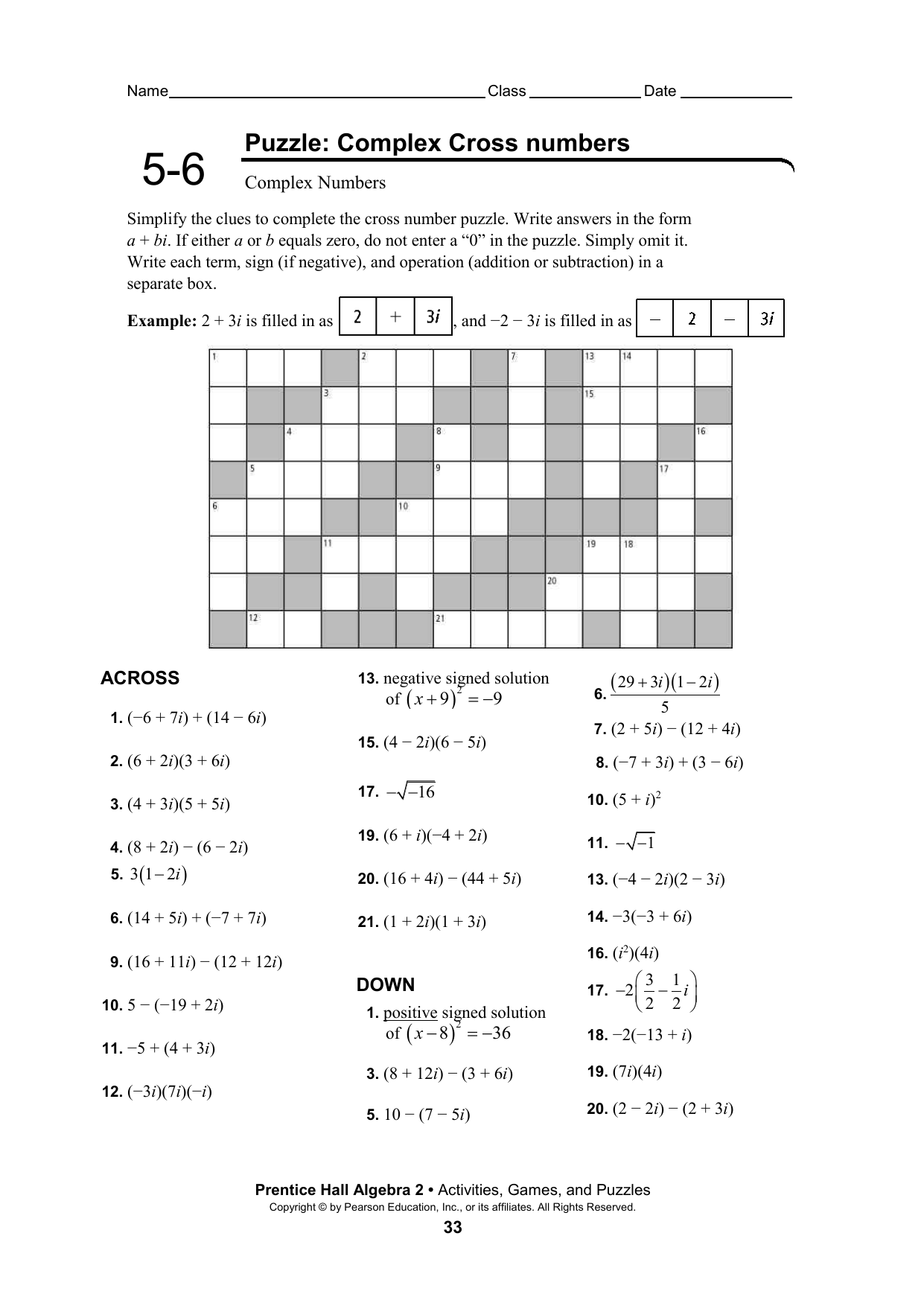
Puzzle: Complex Cross numbers Complex Numbers Simplify the clues to complete the cross number puzzle. Write answers in the form a + bi. If either a or b equals zero, do not enter a '0' in the puzzle. Write each term, sign (if negative), and operation (addition or subtraction) in a separate box. 2 + and —2 3i is filled in as. Write each quotient as a complex number. 3 2 2i 4 2 3i 31. 7 5 2 2i Find the factors of each expression. Check your answer. 24x2 2 49 Find all solutions to each quadratic equation. Number Puzzles Collection of best number puzzles. Number puzzles have specific set of rules, you first has to figure out the pattern being followed and then answer the puzzle according to the pattern. Number puzzles are a part of many competitive examinations, these also helps you improve your logical thinking and above all these are fun to.
2) The A4 clue says that A4, B3, C2, and D1 all have an adjacent cell that contains a larger number. Thus, D1 cannot be 16, as it's the largest number in the puzzle. D1 = 09 and D4 = 16.
3) There are only six prime numbers between 01 and 16, and six open spots in the first two columns. The clue in D1 guarantees that all of the unfilled spots correspond to 02, 03, 05, 07, 11, and 13. But which spots? D4 says that one column lists four consecutive numbers. Column 1 is a candidate. Column 4 is not. Column 2 is not, because there are no four consecutive prime numbers. Column 3 is not, because there are no four consecutive non-prime numbers between 01 and 16. Therefore, Column 1 is the one with consecutive numbers: A2 = 02, and A3 = 03.
4) You learn in A3 that B1 + C1 = 11. What could B1 equal? The remaining primes are 05, 07, 11, and 13. B1 cannot be 11 or 13, because that would make the equation B1 + C1 = 11 impossible. If B1 = 07, then C1 = 04, but this is impossible, because A4 = 04. If B1 = 05, then C1 = 06, which is the only remaining possible solution.
5) Now, C1's clue tells us that 2 + B2 = 4 + B4, or B2 – B4 = 2. The candidates for the B column are 07, 11, and 13. The only pair of numbers in this set whose difference is 2 is 11 (in B4) and 13 (in B2). This leaves B3 = 07 as the only unused prime.
6) The clues in B3 and B4 are new. B4 is nice to know, but not helpful right now. B3 gets you almost to the end, though. Look at the sum of numbers in rows 3 and 4.

Row 3 Sum: 10 + C3 + D3
Row 4 Sum: 31 + C4
The clue in B3 tells us that the Row 3 sum >= the Row 4 Sum. Unused numbers are: 08, 10, 12, 14, 15.
The biggest the Row 3 Sum can be is 10 + 14 + 15 = 39.
Cross Number Puzzle 25
The smallest the Row 4 Sum can be is 31 + 08 = 39.
Complex Number Puzzles Printable
To satisfy this clue, you know that Row 3 Sum = Row 4 Sum = 39.
To summarize: Row 4 gets the 08 (in C4).
Row 3 gets the 14 and 15, although we don't know the order yet.
Row 2 gets the 10 and 12. Because C2 is not 10 (clue B4), then C2 = 12, and D2 = 10. This leaves just two cells.
4-8 Puzzle Complex Cross Numbers Answers
3) There are only six prime numbers between 01 and 16, and six open spots in the first two columns. The clue in D1 guarantees that all of the unfilled spots correspond to 02, 03, 05, 07, 11, and 13. But which spots? D4 says that one column lists four consecutive numbers. Column 1 is a candidate. Column 4 is not. Column 2 is not, because there are no four consecutive prime numbers. Column 3 is not, because there are no four consecutive non-prime numbers between 01 and 16. Therefore, Column 1 is the one with consecutive numbers: A2 = 02, and A3 = 03.
4) You learn in A3 that B1 + C1 = 11. What could B1 equal? The remaining primes are 05, 07, 11, and 13. B1 cannot be 11 or 13, because that would make the equation B1 + C1 = 11 impossible. If B1 = 07, then C1 = 04, but this is impossible, because A4 = 04. If B1 = 05, then C1 = 06, which is the only remaining possible solution.
5) Now, C1's clue tells us that 2 + B2 = 4 + B4, or B2 – B4 = 2. The candidates for the B column are 07, 11, and 13. The only pair of numbers in this set whose difference is 2 is 11 (in B4) and 13 (in B2). This leaves B3 = 07 as the only unused prime.
6) The clues in B3 and B4 are new. B4 is nice to know, but not helpful right now. B3 gets you almost to the end, though. Look at the sum of numbers in rows 3 and 4.
Row 3 Sum: 10 + C3 + D3
Row 4 Sum: 31 + C4
The clue in B3 tells us that the Row 3 sum >= the Row 4 Sum. Unused numbers are: 08, 10, 12, 14, 15.
The biggest the Row 3 Sum can be is 10 + 14 + 15 = 39.
Cross Number Puzzle 25
The smallest the Row 4 Sum can be is 31 + 08 = 39.
Complex Number Puzzles Printable
To satisfy this clue, you know that Row 3 Sum = Row 4 Sum = 39.
To summarize: Row 4 gets the 08 (in C4).
Row 3 gets the 14 and 15, although we don't know the order yet.
Row 2 gets the 10 and 12. Because C2 is not 10 (clue B4), then C2 = 12, and D2 = 10. This leaves just two cells.
4-8 Puzzle Complex Cross Numbers Answers
7) Column 3 has no odd numbers, but C4 says that every column must have an odd number. So 15 goes in C3, and 14 goes in D3.
